Discussion :: Alligation or Mixture
-
A milk vendor has 2 cans of milk. The first contains 25% water and the rest milk. The second contains 50% water. How much milk should he mix from each of the containers so as to get 12 litres of milk such that the ratio of water to milk is 3 : 5?
Whatsapp
Facebook
Answer : Option B
Explanation :
Let the cost of 1 litre milk be Re. 1
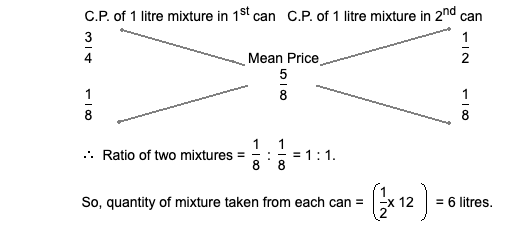
Milk in 1 litre mix. in 1st can = \(\frac { 3 } { 4 } \) litre, C.P. of 1 litre mix. in 1st can Re. \(\frac { 3 } { 4 } \)
Milk in 1 litre mix. in 2nd can = \( \frac { 1 } { 2 }\) litre,C.P. of 1 litre mix. in 2 nd can Re. \( \frac { 1 } { 2 }\)
Milk in 1 litre of final mix. = \( \frac { 5 } { 8 }\) litre, Mean price = Re. \( \frac { 5 } { 8 }\)
By the rule of alligation, we have
Be The First To Comment