Discussion :: Area
-
The percentage increase in the area of a rectangle, if each of its sides is increased by 20% is:
Whatsapp
Facebook
Answer : Option C
Explanation :
Let original length = x metres and original breadth = y metres.
Original area = (xy) m2.
New length=\( [\frac { 120 } { 100 } \)x]m=\([ \frac {6 } { 5 }X ]\)m
New breadth =\( [\frac { 120 } { 100 } \)y]m=\([ \frac {6 } { 5 }Y ]\)m
New Area =[\( \frac {6 } { 5 }XX \)\( \frac {6 } { 5 }Y ]\)\( m^2\)=\([ \frac {36 } {2 5 }XY ]\)\( m^2\)
The difference between the original area = xy and new-area 36/25 xy is
= (36/25)xy - xy
= xy(36/25 - 1)
= xy(11/25) or (11/25)xy
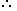Increase % =[\( \frac {11 } { 25 }XY \)x\( \frac { 1 } { XY } \)x100]%=44%
Be The First To Comment