Discussion :: Banker's Discount
-
The banker's gain on a certain sum due 1 12 years hence is 325 of the banker's discount. The rate percent is:
Answer : Option B
Explanation :
Let, B.D = Re. 1.
Then, B.G. = Re .325
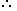 T.D. = (B.D. - B.G.) = Re [1-325 ]= Re.2225
T.D. = (B.D. - B.G.) = Re [1-325 ]= Re.2225
Sum = [[1∗(22/25)1−(22/25)] =Rs.223
S.I. on Rs. 223 for 1 12yeras is Re .1.
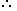 Rate = [100∗122/3∗3/2]% = 10011 = 9 111%
Rate = [100∗122/3∗3/2]% = 10011 = 9 111%
Be The First To Comment


 Whatsapp
Whatsapp
 Facebook
Facebook

