Discussion :: Pipes and Cistern
-
Two pipes A and B can fill a tank in 15 minutes and 20 minutes respectively. Both the pipes are opened together but after 4 minutes, pipe A is turned off. What is the total time required to fill the tank?
Answer : Option D
Explanation :
Part filled in 4 minutes =4 [115+120] =715
Remaining part =[1- 715]=815
Part filled by B in 1 minute =120
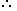 120:815::1:x
120:815::1:x
x =[815x1 x20]=10 23 min = 10 min. 40 sec.
 The tank will be full in (4 min. + 10 min. + 40 sec.) = 14 min. 40 sec.
The tank will be full in (4 min. + 10 min. + 40 sec.) = 14 min. 40 sec.
Be The First To Comment


 Whatsapp
Whatsapp
 Facebook
Facebook

