Discussion :: Probability
-
In a class, there are 15 boys and 10 girls. Three students are selected at random. The probability that 1 girl and 2 boys are selected, is:
Answer : Option A
Explanation :
Let S be the sample space and E be the event of selecting 1 girl and 2 boys.
| Then, n(S) | = Number ways of selecting 3 students out of 25 |
| = 25C3 |
=(25∗24∗23)(3∗2∗1)
=2300
| n(E) |
= (10C1 x 15C2) |
= [ 10 X (15∗14)(2∗1)]
=1050
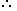 P(E) = n(E)n(S)= 10502300=2146
P(E) = n(E)n(S)= 10502300=2146
Be The First To Comment


 Whatsapp
Whatsapp
 Facebook
Facebook

