Discussion :: Simplification
-
A fires 5 shots to B's 3 but A kills only once in 3 shots while B kills once in 2 shots. When B has missed 27 times, A has killed:
Whatsapp
Facebook
Answer : Option A
Explanation :
Let the total number of shots be x. Then,
shots fired by A= \(\frac { 5 } { 8 } X\)
shots fired byB=\(\frac { 3 } { 8 } X\)
Killing shots by A =\( \frac { 1 } { 3 } \)of\(\frac { 5 } { 8 } X\)=\( \frac { 5} { 24 }X\)
Shots missed by B =\( \frac { 1 } { 2 }\)of\(\frac { 3 } { 8 } X\)=\( \frac { 3 } { 16 }X \)
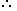\( \frac { 3X } { 16 } \)=27or X=\([\frac { 27*16 } {3}]\)=144
Birds killed by A =\(\frac { 5X} { 24 }\)=\( [ \frac { 5 } { 24 }*144] \)=30
Be The First To Comment