Discussion :: Square Root and Cube Root
-
If x =√3+1√3−1 and Y = √3−1√3+1 ,then the value of (x2 + y2) is:
Whatsapp
Facebook
Answer : Option C
Explanation :
X = (√3+1)(√3−1) x (√3+1)(√3+1) = (√3+1)2(3−1) = 3+1+2√32 = 2+√3
y = (√3−1)(√3+1) x (√3−1)(√3−1) = (√3−1)2(3−1)= 3+1−2√32= 2 -√3
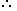x2 + y2 = (2 +√3 )2 + (2 - √3)2
= 2(4 + 3)
= 14
Be The First To Comment