Answer : Option D

Explanation :

Statement (d) is false because a finite-duration sequence must have an ROC that includes the entire z-plane, except possible z = 0 and/or z = ∞

This is not consistent with having a pole at z = 1/2.

Statement (b) is true corresponds to the value of the z-transform of h[n] at z = 2.

Thus, its convergence is equivalent to the point z = 2 being in the ROC.

Since the system is stable and causal, all of the poles of H(z) are inside the unit circle, and the ROC includes all the points outside the unit circle, including z = 2.

Statement (c) is true. Since the system is causal, h[n] = 0 for n < 0.

Consequently, h[n] * h[n] = 0 for n < 0; i.e., system with h[n] * h[n] as its impulse response is causal.

The same is then true for g[n] = n[h[n]].

Furthermore, by the convolution property the system function corresponding to the impulse response h[n] * h[n] is H²(z), and by the differentiation property the system function corresponding to g[n] is

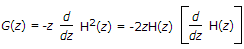
From above equation we can conclude that the poles of G(z) are at the same locations as those of H(z), with the possible exception of the origin.

Therefore, since H(z) has all its poles inside the unit circle, so must G(z).

It follows that g[n] is the impulse response of a causal and stable system.

Statement (a) is true because there is a zero on the unit circle.