Menu
Home / ECE / Exam Questions Paper :: Discussion

Discussion :: Exam Questions Paper

  1. Find the Fourier transform of the half cosine pulse as shown below :

    2. A.
    0.5 {sin c [0.25(f - 1)] + sin c [2.5(f + 1)]}
    B.
    0.5 {sin c [0.5(f - 1)] + sin c [0.5(f + 1)]}
    C.
    0.25 {sin c [0.25(f - 1)] + sin c [0.25 (f - 1)]}
    D.
    0.25 {sin c [0.5(f - 1)] + sin c [0.5(f - 1)]}

    View Answer

    Workspace

    View Answer

    Answer : Option B

    Explanation :

    The given signal can be expressed as multiplication of x1(t) and x2(t) as shown below.

    where A = 2, T/2 = 0.25 => T = 0.5

    ∴ x(t) = x1(t) x x2(t)

    => X(f) = X1(f) * X2(f)

    Now X1(f) = 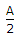[δ(f - f0) + δ(f + f0)]

    = [sin c [T(f - f0)] + sin c[T(f + f0)]]

    Now, A = 2, T = 0.5 and f0 = = 1

    => X(f) = 0.5[sin c (0.5(f - 1)) + sin c (0.5(f + 1))].

Write your comments here:

Be The First To Comment