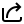Answer : Option C
Explanation :
Solution 1:
We have 0.3̅4̅2̅1 = 0.342134213421........ (i)
Multiply both sides by 10, we get
10 * 0.3̅4̅2̅1 = 3.421................... (ii)
But on comparing both equations, the repeating number should be the same after the decimal point.
So, multiply equation 2 by 1000, we get
10000* 0.3̅4̅2̅1 = 3421.342134213421.... ....... (iii)
Now subtract equation i from iii, we get
(10000 - 1) * 0.3̅4̅2̅1 = 3421.342134213421... - 0.342134213421.....
Or, 9999 * 0.3̅4̅2̅1= 3421
Or, 0.3̅4̅2̅1 = 3421/9999
Solution 2:
Quick method:
Note: the period of 0.7̅ is 7, so the numerator of the fraction is 7, and there is one digit in the period, the denominator will have one nine.
Therefore, the vulgar fraction = 7/9
Similarly, the period of 0.3̅4̅2̅1 is 3421, so the numerator of the fraction is 3421, and there is four digit in the period, the denominator will have four nine.
Hence, the vulgar fraction will be 3421/9999.


 Whatsapp
Whatsapp
 Facebook
Facebook