Loading [MathJax]/jax/output/CommonHTML/jax.js
Arithmetic Aptitude :: Surds and Indices
Answer : Option D
Explanation :
Let (17)3.5 x (17)x = 178.
Then, (17)3.5 + x = 178.
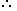 3.5 + x = 8
3.5 + x = 8
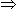 x = (8 - 3.5)
x = (8 - 3.5)
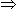 x = 4.5
x = 4.5
-
If[ab]X−1=[ba]X−3, then the value of x is
Answer : Option C
Explanation :
Given [ab]X−1= [ba]X−3
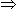 [ab]X−1=[ab](X−3)=[ab](3−X)
[ab]X−1=[ab](X−3)=[ab](3−X)
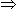 x - 1 = 3 - x
x - 1 = 3 - x
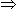 2x = 4
2x = 4
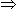 x = 2.
x = 2.
-
Given that 100.48 = x, 100.70 = y and xz = y2, then the value of z is close to:
Answer : Option C
Explanation :
xz = y2  10(0.48z) = 10(2 x 0.70) = 101.40
10(0.48z) = 10(2 x 0.70) = 101.40
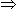 0.48z = 1.40
0.48z = 1.40
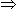 z =14048=3512=2.9(approx.)
z =14048=3512=2.9(approx.)
-
If 5a = 3125, then the value of 5(a - 3) is:
Answer : Option A
Explanation :
5a = 3125  5a = 55
5a = 55
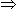 a = 5.
a = 5.
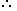 5(a - 3) = 5(5 - 3) = 52 = 25.
5(a - 3) = 5(5 - 3) = 52 = 25.
-
If 3(x - y) = 27 and 3(x + y) = 243, then x is equal to:
Answer : Option C
Explanation :
3x - y = 27 = 33  x - y = 3 ....(i)
x - y = 3 ....(i)
3x + y = 243 = 35  x + y = 5 ....(ii)
x + y = 5 ....(ii)
On solving (i) and (ii), we get x = 4.
-
|
(256)0.16 x (256)0.09 = ?
|
Answer : Option A
Explanation :
(256)0.16 x (256)0.09 = (256)(0.16 + 0.09)
= (256)0.25
= (256)(25/100)
= (256)(1/4)
= (44)(1/4)
= 44(1/4)
= 41
= 4
-
The value of [(10)150 ÷ (10)146]
Answer : Option B
Explanation :
= 10150 - 146
= 104
= 10000.
| (10)150 ÷ (10)146 =1015010146 |
-
(25)7.5 x (5)2.5 ÷ (125)1.5 = 5?
Answer : Option B
Explanation :
Let (25)7.5 x (5)2.5 ÷ (125)1.5 = 5x.
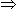 Then,(52)*(5)2.5/(5^3)^1.5=5X
Then,(52)*(5)2.5/(5^3)^1.5=5X
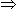 5(2∗7.5)∗52.55(3∗1.5)=5X
5(2∗7.5)∗52.55(3∗1.5)=5X
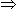 5x = 5(15 + 2.5 - 4.5)
5x = 5(15 + 2.5 - 4.5)
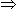 5x = 513
5x = 513
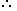 x = 13.
x = 13.
-
11+X(b−a)+X(c−a)+11+X(a−b)+X(c−b)+11+X(b−c)+X(a−c)=?
Answer : Option B
Explanation :
Given Exp =1(1+xbxa+xcxa)+1(1+xaxb+xcxb)+1(1+xbxc+xaxc)
=Xa(Xa+Xb+Xc)+Xb(Xa+Xb+Xc)+Xc(Xa+Xb+Xc)
=(Xa+Xb+Xc)€‹€‹(Xa+Xb+Xc)
=1
Answer : Option B
Explanation :
(0.04)-1.5 =[4100]−1.5
=[125]−(3/2)
= (25)(3/2)
= (52)(3/2)
= (5)2 x (3/2)
= 53
= 125.
Or drag and drop files below